The laser refraction tank consists of a laser (I suppose that part should be obvious) and a tank (which I suppose should also be at least as obvious). The tank has angle markings, as shown, and a hole on top so that it may be partially filled with a liquid.
If the tank is filled halfway with, say, water, then the plane to which all of the angles are measured against becomes an interface between two regions of different index of refraction n. Air has an index of refraction close to n = 1 and water has an index of refraction of about n = 1.33, give or take a couple hundredths based on temperature, pressure, fish poop, etc.. When the laser is shined in from above, it passes into a region of higher index of refraction at the interface, and thus the light bends toward the normal (imaginary line drawn perpendicular to the interface), as determined by Snell's La:. n1sinθ1=n2sinθ2, where the angles θ1 and θ2 are measured with respect to the normal.
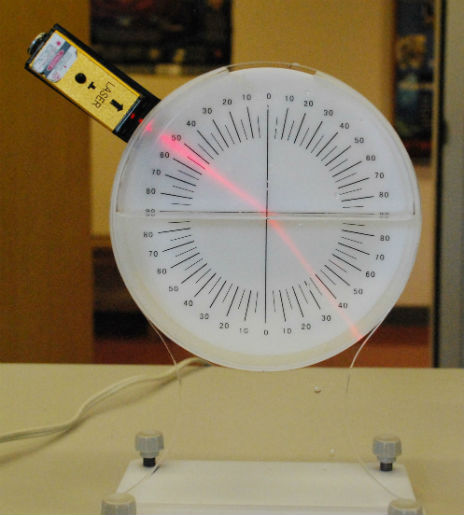
From beneath, the light bends away from the normal.
Light incident on a region of decreased index of refraction has another interesting consequence: it reflects. Since the light bends away from the normal as it passes into a region of lower index of refraction, it is easy to imagine a point where the refracted light will be exactly parallel to the surface of the water. But what happens if the laser is moved beyond that angle? At that point, it is impossible for any light to pass into the region of lower index of refraction because the angle is too steep, and thus we have total internal reflection.
